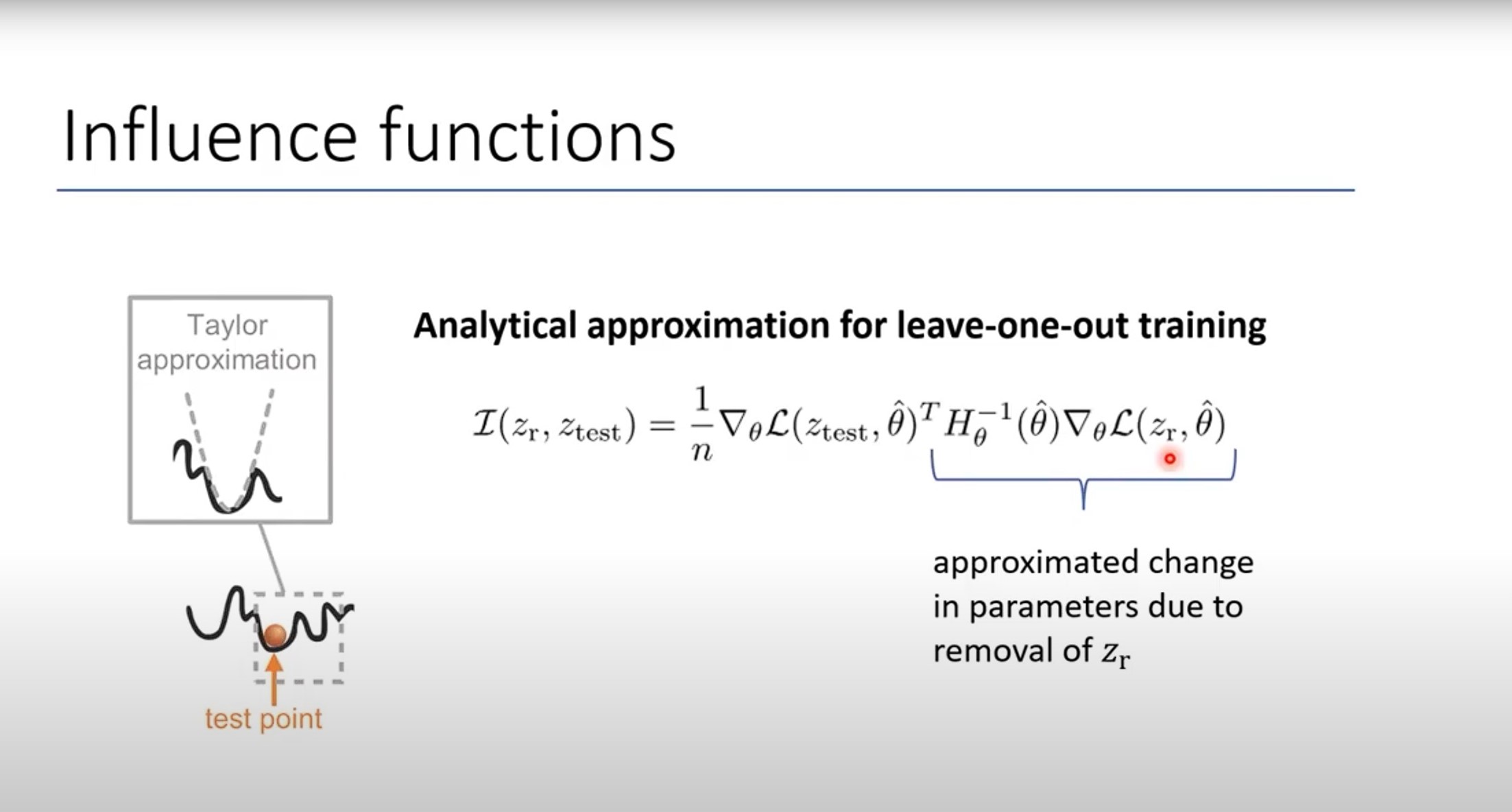
Marcin Łobejko (University of Gdansk): Towards reconciliation of completely positive open system dynamics with equilibration postulate

Quantum Information and Quantum Computing Seminars CTP PAS

Almost every quantum system interacts with a large environment, so the exact quantum mechanical description of its evolution is impossible. One has to resort to approximate description, usually in the form of a master equation. There are at least two basic requirements for such description: first of all, it should preserve positivity of probabilities; second, it should reproduce the wisdom coming from thermodynamics - systems coupled to a single thermal bath tend to the equilibrium. Existing two widespread descriptions of evolution fail to satisfy at least one of those conditions. The so-called Davies master equation, while preserving positivity of probabilities (due to Gorini-Kossakowski-Sudarshan-Lindblad form), fails to describe thermalization properly. On the other hand, the Bloch-Redfield master equation violates the positivity of probabilities, but it correctly describes equilibration at least for off-diagonal elements for several important scenarios. However, is it possible to have a description of open system dynamics that would share both features? In this talk, I will show our recent research that partially resolves this problem. (i) We provide a general form, up to second order, of the proper thermal equilibrium state (which is nontrivial even in the weak coupling limit). (ii) Next, we derive the steady-state coherences for a whole class of master equations, and in particular, we show that the solution for the Bloch-Redfield equation coincides with the equilibrium state. (iii) We consider the so-called cumulant equation, which is explicitly completely positive, and we show that up to second order, its steady-state coherences are the same as one of the Bloch-Redfield dynamics. (iv) We solve the second-order correction to the diagonal part of the stationary-state for a two-level system for both the Bloch-Redfield and cumulant master equation, showing that the solution of the cumulant is very close to the equilibrium state, whereas the Bloch-Redfield differs significantly.