Julio de Vicente (Universidad Carlos III de Madrid): Genuine multipartite entanglement and nonlocality in pair-entangled network states
Quantum Information and Quantum Computing Seminars CTP PAS
The study of entanglement and nonlocality in multipartite quantum states plays a major role in quantum information theory and genuine multipartite entanglement (GME) and nonlocality (GMNL) signal some of its strongest forms for applications. However, their characterization for general (mixed) states is a highly nontrivial problem and its experimental preparation faces the formidable challenge of controlling quantum states with many constituents. In this talk I introduce a subclass of multipartite states, which I term pair-entangled network (PEN) states, as those that can be created by distributing exclusively bipartite entanglement in a connected network, and I study how their entanglement and nonlocality properties are affected by noise and the geometry of the graph that provides the connection pattern. The motivation is twofold. First, this class represents arguably the most feasible way to prepare GME and GMNL states in practice. Second, the class of PEN states provides an operationally motivated subset of multipartite states in which the well-developed theory of bipartite entanglement can be exploited to analyze entanglement in the multipartite scenario. I will show that all pure PEN states are GME and GMNL independently of the amount of entanglement shared and the network (as long as it is connected). In contrast, in the case of mixed PEN states these properties depend both on the level of noise and the network topology and they are not guaranteed by the mere distribution of mixed bipartite entangled states. In particular, the amount of connectivity in the network determines whether GME is robust to noise for any system size or whether it is completely washed out under the slightest form of noise for a sufficiently large number of parties. This latter case implies fundamental limitations for the application of certain networks in realistic scenarios, where the presence of some form of noise is unavoidable. In addition to this, if time allows, to illustrate the applicability of PEN states to study the complex phenomenology behind multipartite entanglement I will present three more results which use them as a proof ingredient: (i) all pure GME states are GMNL in the multiple-copy scenario, (ii) GMNL can be superactivated for any number of parties, and (iii) the set of GME-activatable states can be characterized as those states that are not partially separable.
Other seminars

Giulio Chiribella (University of Hong Kong): Optimal programming of quantum gates - Duplicate27-06-2022

Marco Tomamichel (National University of Singapore): Quantum advantage with noisy shallow circuits17-05-2022

R. Patron (University of Edinburgh): Limitations of optimization algorithms on noisy quantum devices02-03-2022

Marcel Hinsche (FU Berlin): Learnability of the output distributions of local quantum circuits25.05.2022

Beata Zjawin (ICTQT): Inequality Constraints in Directed Acyclic Graphs with Hidden Variables02.02.2022

Laura Mancinska (QMATH, Copenhagen): Fixed-size schemes for certification of large quantum systems17-11-2021
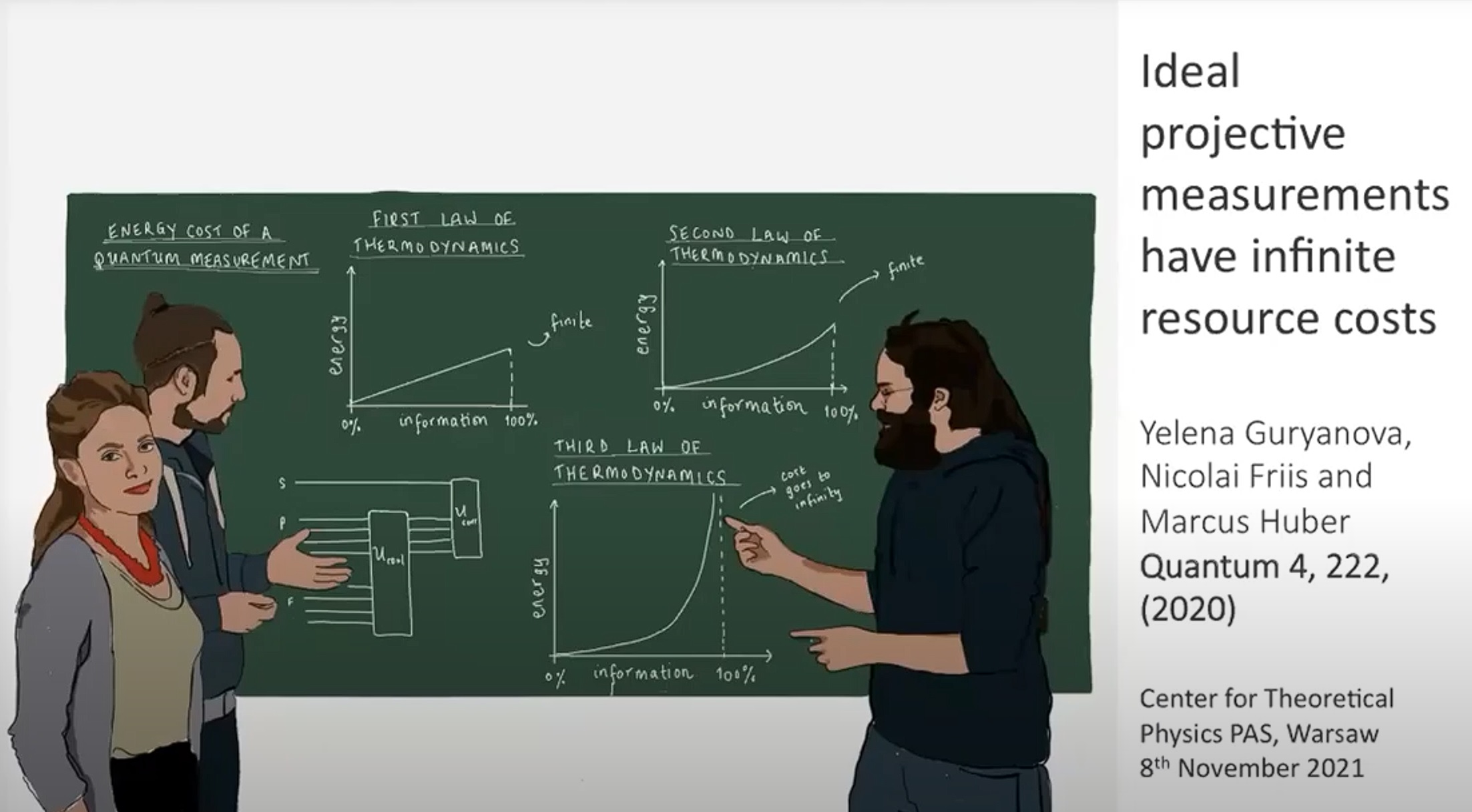
Yelena Guyanova (IQOQI, Vienna): Ideal Projective Measurements Have Infinite Resource Costs10-11-2021

Hamed Mohammady (ULdB): Measurement disturbance and conservation laws in quantum mechanics03-11-2021